There are different ways to calculate the quality of a laser beam. In general, we want our laser beam to be as close to a theoretical Gaussian Beam as possible. The question then is how do we measure the profile of our beam and how do we compare it against our ideal Gaussian Beam?
First, we need to understand that there are different ways to calculate the quality of a laser beam. Some of the ways to characterize a laser beam are: beam parameter product, Strehl ratio, and beam quality factor (or M-squared). It is the last one that we want to talk about today.
M-squared Factor
The M-squared factor is useful when we have laser beams that need to be focused. We are basically comparing the divergence and beam waist size to that of a Gaussian Beam.
The M-squared factor is defined by the ISO Standard 11146 as:
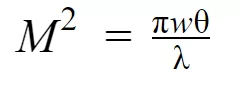
Where w is the beam waist, θ is the divergence angle, and ? is the laser wavelength. Furthermore, if we assume that the divergence of a Gaussian beam is given by:
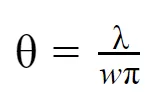
Divergence Equation
It’s easy to show that for a perfect Gaussian beam, the M-squared factor will have a value of 1. The larger the M-squared factor is, the farther away from a Gaussian beam our laser beam will be.
Measuring M-squared Factor
In order to calculate the M-squared factor, you will need at least two measurements. This makes it possible to estimate the beam divergence and waist. However, the ISO 11146 norm explains a method to calculate M2 from a set of 10 beam diameter measurements. The main steps are:
- Start with a collimated beam
- Focus it with an aberration-free lens
- Measure the diameter of the beam at various positions around the focal point: take at least 10 data points, approximately half within the first Rayleigh distance of the focal point, and the other half beyond 2 Rayleigh lengths.
- Use the regression equations detailed in the norm to fit a hyperbola to your data points for both the X and Y axes.
- From this fit, extract the values for θ, w0, zR, and M2 for each axis.
This measurement can be made using the knife-edge method or the scanning slit measurement. These measurements can be time consuming, so it’s better if the sensor is placed on a motorized translation stage to automate this process.
Free Tools
We have developed an open source tool that will help you analyze your beam profile. You can work in either a static mode where you upload your beam image, or in a dynamic mode where the software will give measurements in real time. We have also developed the mechanical setup to measure beam divergence.
The measurements obtained by the software can help you quantify your laser quality, or redesign the optical components to obtain a particular spot beam for your system. The software also allows you to export your data to an excel worksheet (along with the graphs)
The laser profile tool can be found HERE
A perfect example of the use of M-squared parameters is when using laser beam homogenizers.
If you are unsure if the M-squared factor is the right beam parameter that you need for your application, give us a call and we can advise you.
Learn more about the unique properties of lasers in these recent posts Laser Rayleigh Range: A Detailed Explanation and Laser Beam Caustic: Explanied.